Sketch the Graph of the Function

Use the y intercept x intercepts and other properties of the graph of to sketch the graph of f. Simple families of rational function produce mesmerizing animations.

Can You Match All The Graphs With Their Respective Derivatives Like You Did In Class This Is The Alternate Homework A Ap Calculus Math Manipulatives Calculus

For the basic function its basic graph is just a parabola.

. Plot at least 5 Points each. Y3x1 is a linear function. Here are some simple things we can do to move or scale it on the graph.

When done the frames will be antialiased and animated at 24 fps. Graphing Quadratics - PhET. Find the horizontal asymptote.

This new function in fact is called the derivative of the original function. The equation for this graph will be in the form where A is the amplitude f is the frequency h is the horizontal shift and k is the vertical shift. The distance between the maximum and the minimum.

A graph of a function is a visual representation of a functions behavior on an x-y plane. So the graph of a cube function may have a maximum of 3 roots. Start with basic shapes.

Demonstrates Arduinos advanced serial output functions. A rough blurry sketch is drawn quickly and finer-grained rendering will follow for several minutes. We can do this in two ways.

We can make a table of values or we can interpret this as a transformation. It cannot have 2 real zeros. Please contact Savvas Learning Company for product support.

The line graph is used to solve changin g conditions often over a certain time interval. A general linear function has the form y mx c where m and c are constants. Fx x 2.

Using a dashed or lightly drawn line. Let us start with a function in this case it is fx x 2 but it could be anything. A line graph is a graph formed by segments of straight lines that join the plotted points that represent given data.

From sketching the maximum and the minimum we can see that the graph is centered at and has an amplitude of 2. For large positive or negative values of x 178x 4 approaches zero and the graph approximates the line y 12x - 74. Beyond simple math and grouping like x2x-4 there are some functions you can use as well.

Its graph would be a parabola. From plotting the maximum and minimum we can see that the graph is centered on with an amplitude of 3. Choose a set of points from the graph of f x x3 to work with.

2 Identify the exponential function. If the original is referred to. The rules from graph translations are used to sketch the derived inverse or other related functions.

Its graph would be a hyperbola. A - The y intercept is given by 0 f0 0 8 b - The zeros of f are solutions to - x - 2 3 0 Function f has one zero at x 2 of multiplicity 3 and therefore the graph of f cuts the x axis at x 2. Sketch the graph of the function by first making a table of values.

It has x as the exponent and the base is 3 which is greater than 1. Sketch the graph of the function by first making a table of values. If an answer is undefined enter UNDEFINED Ct 1 t 1.

Sketch the graph of the secant equation y sec x - π4. Yx23 is a quadratic function. Since complex roots always occur in pairs a cubic function always has either 1 or 3 real zeros.

C 0 moves it down. Solution to Example 2. First identify the parameters before sketching the trigonometric secant graph.

The distance from the maximum to the minimum is half the wavelength. With our money back guarantee our customers have the right to request and get a refund at any stage of their order in case something goes wrong. F x x3.

To move the line down we use a negative value for C. To write this equation it is helpful to sketch a graph. Y3x is an exponential function.

Long divide the denominator into the numerator to determine the behavior of y for large absolute values of xIn this example division shows that y 12x - 74 178x 4. If an answer is undefined enter UNDEFINED Ct 1 t 1. Its graph would be a straight line.

For more on MaxMSP see Cycling 74. 1 f x. The basic graph can be looked at as the foundation for graphing the actual function.

This blog will help students graph absolute value graphs apply function transformations and graphing absolute value equations using multiple solved examples. To write the equation it is helpful to sketch a graph. Move the mouse to change the brightness of an LED.

Sketch the graph of each function. This is a function. Identify the range of the given secant equation.

Complete the square to find turning points and find expression for composite functions. The equation will be in the form where A is the amplitude f is the frequency h is the horizontal shift and k is the vertical shift. Since our function has an x3 in it we will use the library function.

A 3 1 4 hx x b gx x 3 Solution a. One trick that can help even the most artistically challenged to create a clearly recognizable basic sketch is in nearly all learn to draw courses. Because more than 300 frames are computed parameterized expressions can take a long time to fully render.

We can move it up or down by adding a constant to the y-value. Draw the graph point for each pair. Sketching the Secant Graph With Horizontal Shift.

The basic graph is exactly what it sounds like the graph of the basic function. To be able to graph a secant function see the examples given below. Yfrac3x is a reciprocal function.

Send data to the computer and graph it in Processing. The basic graph will be used to develop a sketch of the function with its transformations. Sketch the graph of each function.

Starting with one function ds sqrt625-x2 we have derived by means of some slightly nasty algebra a new function ds -x sqrt625-x2 that gives us important information about the original function. Look below to see them all. Ie it may intersect the x-axis at a maximum of 3 points.

The function 3 1 4. There are also MaxMSP patches that can communicate with each Arduino sketch as well. Send MIDI note messages serially.

A cubic function is a polynomial function of degree 3. We know what the basic graph should look like so we just need to understand how the factor of frac 1 2 is going to affect things. Gx x 2 C.

Graphs help us understand different aspects of the function which would be difficult to understand by just looking at the function itself. Simply sketch imaginary lines vertically for each x axis value and. C 0 moves it up.

They are mostly standard functions written as you might expect.

Solving A Rational Function And Graphing It

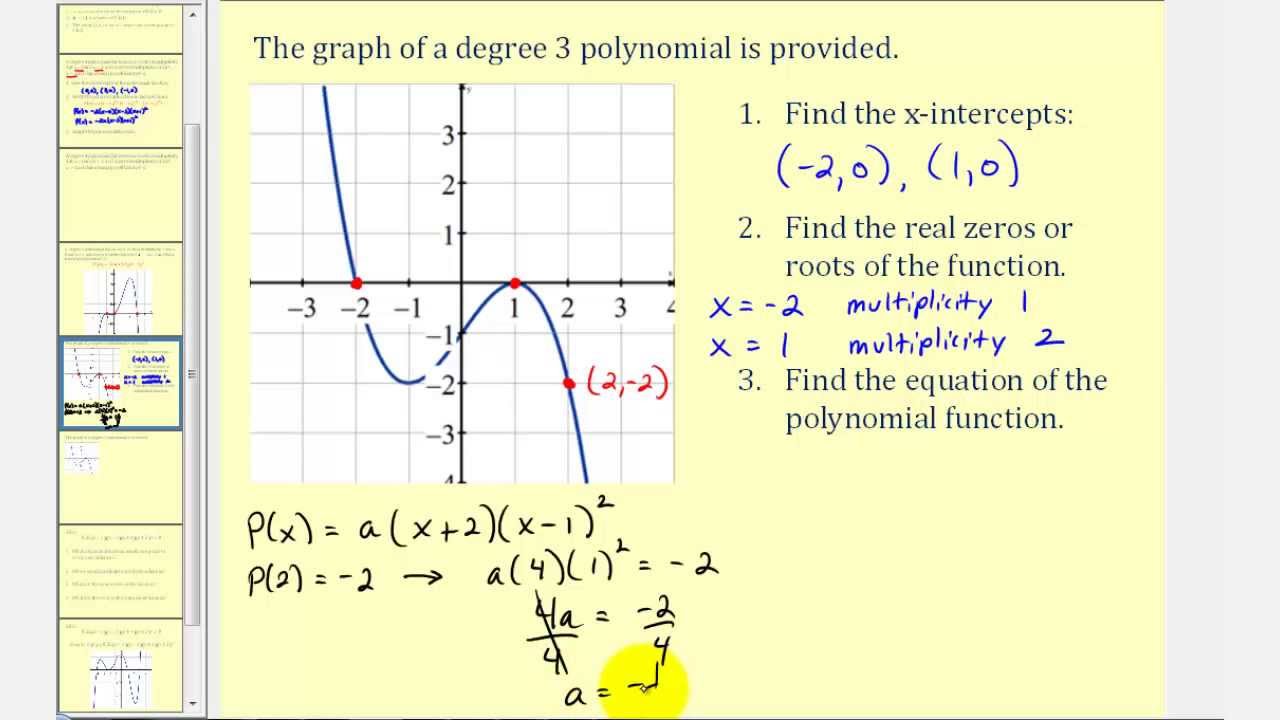
Real Zeros Factors And Graphs Of Polynomial Functions Polynomial Functions Polynomials Graphing Linear Equations Activities

Example 4 Graph A Translated Square Root Function Graph Y 2 X 3 2 Then State The Domain And Range Solution Step Graphing Quadratics Function Of Roots

Step By Step Instructions Of How To Graph The Sine Function Graphing Trigfunction Trigonometry S Math Materials Graphing Quadratics Math Graphic Organizers